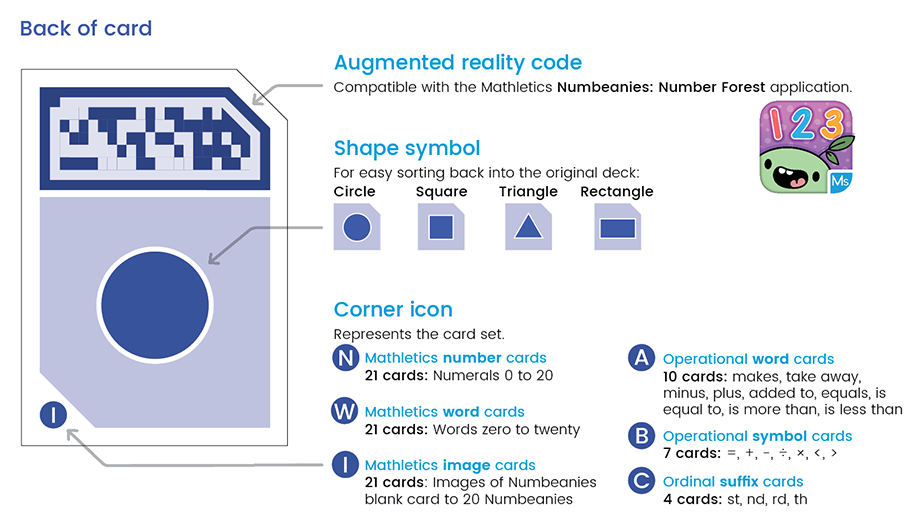
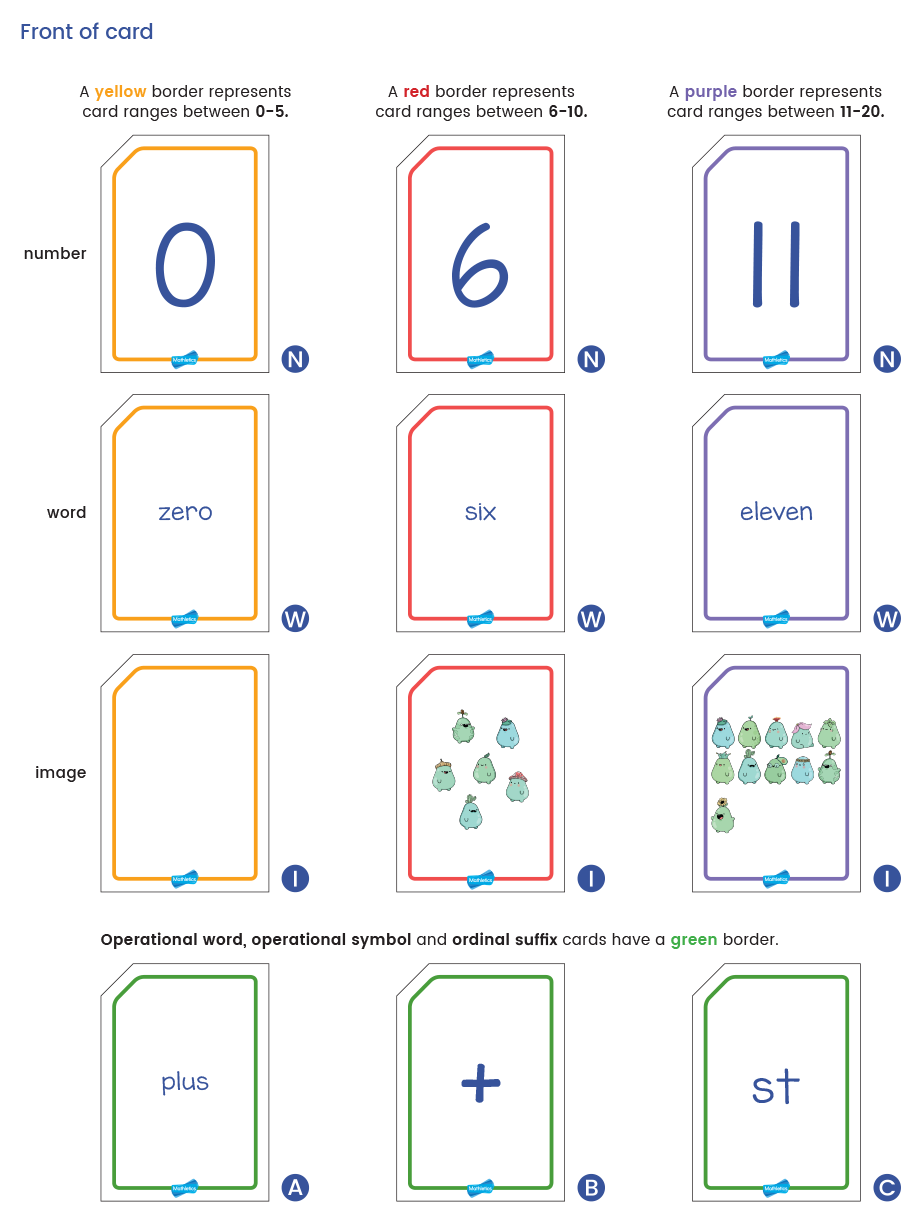
Back to card games
Card games
Number line up
Strand NumberTopic Compare and order
- 0
- Prep < 5 mins

- Play < 5 mins

 Groups
Groups Whole class
Whole class
Summary
A numerical take on the classic game Celebrity Heads. Students must work together to find their correct place in the number line without talking or knowing their own number.
Curriculum content
- Compare and order numbers up to 10
- Read and represent numbers up to 10
Materials
Card sets
- 1 set of Mathletics number cards 1 to 10 (or up to 20)
 1-10
1-10 - 1 set of Mathletics word cards 1 to 10 (or up to 20) or Mathletics image cards 1 to 10 (or up to 20)
 1-10
1-10 1-10
1-10 - Zero can be added to the game.

Concrete
- Plenty of space to move and arrange students in a line
How to play
Set up
- Arrange students into groups of 10 (or up to 20).
- Select cards, eg Mathletics number, word or image cards 1 to 10 (or up to 20).
- Hand out 1 card, face down, to each student.
- Demonstrate placing the card, number facing outwards on your forehead.
- Ask students to do the same without looking at their own card.
- Encourage them to look at the other students and their cards.

Rules
- Instruct players to organize themselves in a line in the right number order, eg ‘Organize yourselves in a line from 1 to 10’.
- Players work together silently to organize their line. No talking!
- Remind players throughout the game not to look at their own card.
- Once players think they are in the correct order the teacher instructs them to look at their own card. Players can then count down the line to check accuracy.
Variations
CHANGE it up
- Use alternative Mathletics word or image cards, or a mixture, to create more of a challenge.
- Vary between counting up and counting down.
- Use a variety of number ranges, skip counts or missing numbers to explore concepts of odds and evens, greater than and less than.
- Play with other group sizes in a competition. Try numerous small teams competing to get the correct order fastest.
- Play with ordinal number cards.
What to look for
- Which students could use number order knowledge to organize themselves and others into the correct position?
- Was there confusion about the direction of the line? How might this be resolved?
- Were students able to establish their own number/position based on the other numbers they saw?
- Which students were unable to determine their own number/position?
- What were the responses of the students who were not involved in the game?